Congruence of Triangles
Congruence of Triangle
Congruence means that one figure will exactly superimpose the other figure. Two congruent line segments have the same length and conversely two lines segments of equal length are congruent. or we can say that two line segments are congruent if and only if their lengths are equal. Similarly two angles are congruent if and only if their measures are equal, or we can say that two angles BAC and EDF are congruent if m
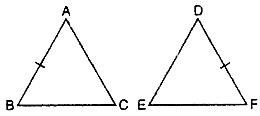
Two triangles are congruent if and only if one of them can be made to superpose on the other, so as to cover it exactly. Let be two congruent triangles. Then, we can superpose
on
, so as to cover it exactly. In such a superposition the vertices of
will fall on the vertices of
, in some order. Let us assume that the vertex A falls on vertex D, vertex B on vertex E and vertex C on vertex F.
Then, side AB falls on DE, BC on EF and CA on FD. Also superposes on the corresponding angle
and
. Thus, the order in which the vertices match, automatically determines a correspondence between the sides and angles of the two triangles. And, if the superposition is exact i.e. the triangles are congruent, the corresponding sides and angles are congruent. Consequently, we get six equalities three of the corresponding sides and three of the corresponding angles. If
superposes on
exactly such that the vertices of
fall on the vertices of
in the following order
|
Then, we have the following six qualities |
 |
Congruence Relation
From the definition of congruence of two triangles, we obtain the following results:
(i) Every triangle is congruent to itself i.e.,
(ii) If , then
(iii) If , and
, then
Note: It is important to write the correspondence of vertices correctly for writing of congruence of triangles in symbolic form because but
and the symbolic form is used to write corresponding parts of congruent triangles {C.P.C.T.}
If | |||
| Right Option : A | |||
| View Explanation | |||
If | |||
| Right Option : A | |||
| View Explanation | |||
Which of the following is not a criteria for congruency ? | |||
| Right Option : D | |||
| View Explanation | |||
Students / Parents Reviews [20]
My experience was very good with Abhyas academy. I am studying here from 6th class and I am satisfied by its results in my life. I improved a lot here ahead of school syllabus.
Ayan Ghosh
8thWhen I have not joined Abhyas Academy, my skills of solving maths problems were not clear. But, after joining it, my skills have been developed and my concepts of science and SST are very well. I also came to know about other subjects such as vedic maths and reasoning.
Sharandeep Singh
7thA marvelous experience with Abhyas. I am glad to share that my ward has achieved more than enough at the Ambala ABHYAS centre. Years have passed on and more and more he has gained. May the centre flourish and develop day by day by the grace of God.
Archit Segal
7thMy experience with Abhyas academy is very good. I did not think that my every subject coming here will be so strong. The main thing is that the online tests had made me learn here more things.
Hiya Gupta
8thIn terms of methodology I want to say that institute provides expert guidence and results oriented monitering supplements by requsite study material along with regular tests which help the students to improve their education skills.The techniques of providing education helps the students to asses...
Aman Kumar Shrivastava
10thMy experience with Abhyas academy is very nice or it can be said wonderful. I have been studying here from seven class. I have been completing my journey of three years. I am tinking that I should join Abhyas Academy in tenth class as I am seeing much improvement in Maths and English
Hridey Preet
9thAbhyas Methodology is very good. It is based on according to student and each child manages accordingly to its properly. Methodology has improved the abilities of students to shine them in future.
Manish Kumar
10thAbhyas is good institution and a innovative institute also. It is a good platform of beginners.Due to Abhyas,he has got knoweledge about reasoning and confidence.My son has improved his vocabulary because of Abhyas.Teacher have very friendly atmosphere also.
Manish Kumar
10thMy experience with Abhyas Academy has been very good. When I was not in Abhyas whenever teacher ask questions I could not speak it confidently but when I came in Abhyas, my speaking skills developed and now I am the first one to give the answer of teachers question.
Upmanyu Sharma
7thAbhyas is an institute of high repute. Yogansh has taken admission last year. It creates abilities in child to prepare for competitive exams. Students are motivated by living prizes on basis of performance in Abhyas exams. He is satisfied with institute.
Yogansh Nyasi
7thAbhyas institute is one of the best coaching institute in the vicinity of Ambala Cantt area. The teachers of the institute are well experienced and very helpful in solving the problems of the students.The good thing of the institute is that it is providing extra classes for the students who are w...
Aman Kumar Shrivastava
10thIt has a great methodology. Students here can get analysis to their test quickly.We can learn easily through PPTs and the testing methods are good. We know that where we have to practice
Barkha Arora
10thAbhyas is a complete education Institute. Here extreme care is taken by teacher with the help of regular exam. Extra classes also conducted by the institute, if the student is weak.
Om Umang
10thAbout Abhyas metholodology the teachers are very nice and hardworking toward students.The Centre Head Mrs Anu Sethi is also a brilliant teacher.Abhyas has taught me how to overcome problems and has always taken my doubts and suppoeted me.
Shreya Shrivastava
8thThird consective year,my ward is in Abhyas with nice experience of admin and transport support.Educational standard of the institute recumbent at satisfactory level. One thing would live to bring in notice that last year study books was distributed after half of the session was over,though study ...
Ayan Ghosh
8thWe started with lot of hope that Abhyas will help in better understnding of complex topics of highers classes. we are not disappointed with the progress our child has made after attending Abhyas. Though need to mention that we expected a lot more. On a scale of 1-10, we would give may be 7.
Manya
8thI have spent a wonderful time in Abhyas academy. It has made my reasoning more apt, English more stronger and Maths an interesting subject for me. It has given me a habbit of self studying
Yatharthi Sharma
10thIt was a good experience with Abhyas Academy. I even faced problems in starting but slowly and steadily overcomed. Especially reasoning classes helped me a lot.
Cheshta
10thMy experience with Abhyas is very good. I have learnt many things here like vedic maths and reasoning also. Teachers here first take our doubts and then there are assignments to verify our weak points.
Shivam Rana
7thAbhyas academy is great place to learn. I have learnt a lot here they have finished my fear of not answering.It has created a habit of self studying in me.The teachers here are very supportive and helpful. Earlier my maths and science was good but now it has been much better than before.
