Congruence of Triangles By SSS Criteria
Congruence of Triangles by SSS Criteria
Theorem: Two triangles are congruence if the three sides of one triangle are equal to the corresponding three sides of the other triangle.
Given: Two such that AB = DE, BC = EF and AC = DF.
To Prove:
Construction: Draw a line segment EG on the other side of EF such that AB = EG and . Join GF and GD.
|
Proof: In BC = EF [Given] AB = GE [By construction] and, So, by SAS criterion of congruence, we have
Now, AB = DE and AB = GE
Similarly, AC = DF and AC = GF In DE = GE ....(iii) In DF = GF [From (ii)]
From (iii) and (iv), we have
But, Thus, in AB = DE [Given] and, AC = DF [Given] So, by SAS criterion of congruence , we have
|
 |
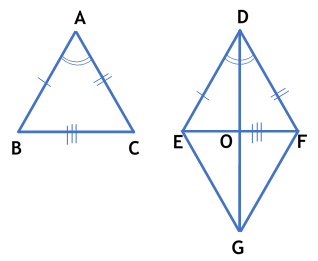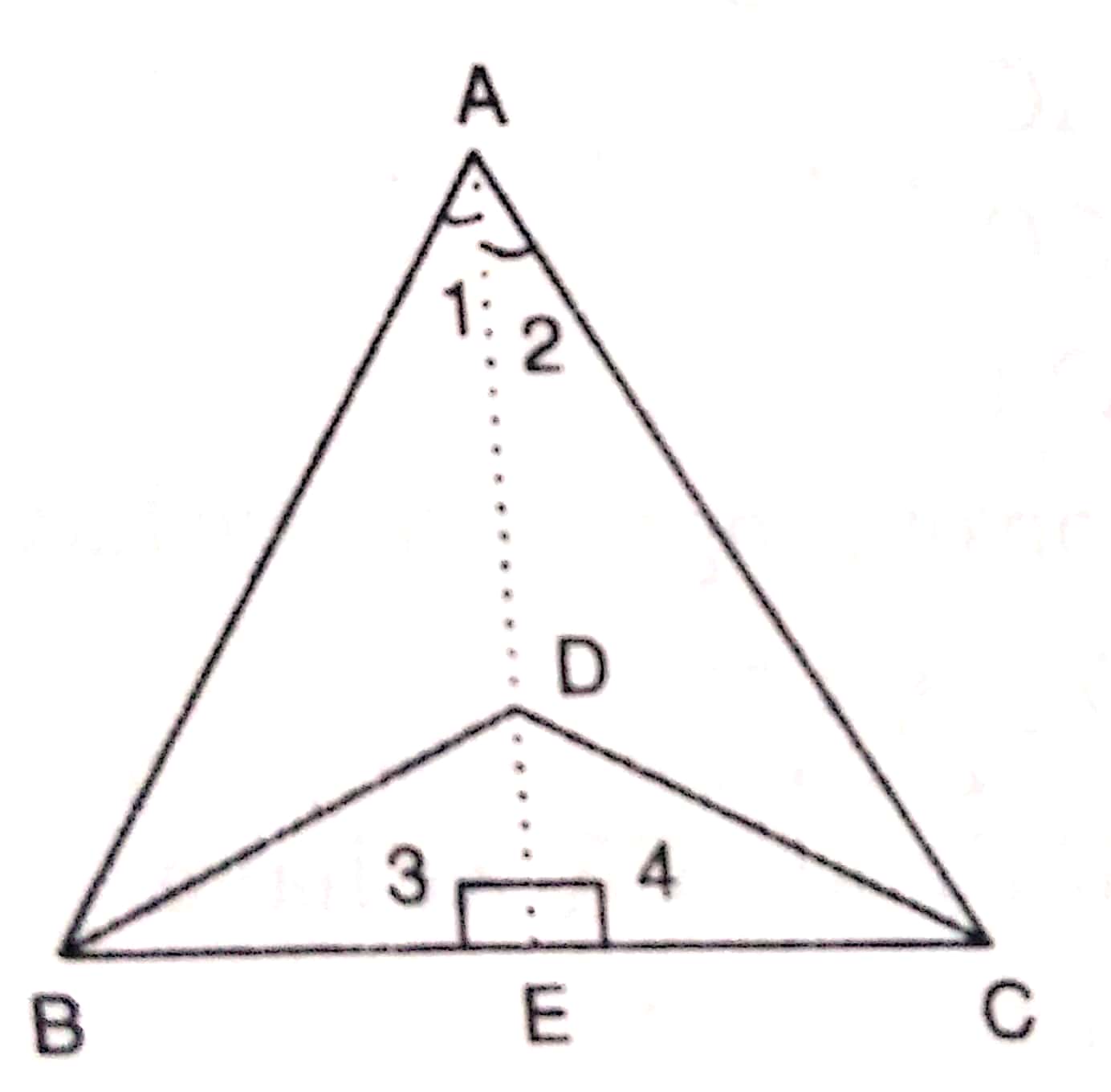
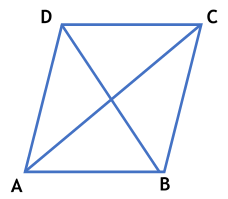
Illustration: ABCD is a parallelogram , if the two diagonals are equal, find the measure of
|
Solution: Since ABCD is a parallelogram. Therefore, AB = CD and AD = BC [ Thus, in AD = BC [As proved above] BD = AC [Given] and, AB = AB [common] So, by SSS criterion of congruence, we have Now,
transversal is Hence, the measure of |
 |
In the figure shown above, if AB = DE, AC = DF and BC = EF, then which two triangles are congruent?
| |||
| Right Option : C | |||
| View Explanation | |||
In the figure below,
| |||
| Right Option : B | |||
| View Explanation | |||
Two isosceles triangles ABC and DBC having the common base BC such that AB = AC and DB = DC. Then
| |||
| Right Option : B | |||
| View Explanation | |||
Students / Parents Reviews [10]
One of the best institutes to develope a child interest in studies.Provides SST and English knowledge also unlike other institutes. Teachers are co operative and friendly online tests andPPT develope practical knowledge also.
Aman Kumar Shrivastava
10thMy experience with Abhyas is very good. I have learnt many things here like vedic maths and reasoning also. Teachers here first take our doubts and then there are assignments to verify our weak points.
Shivam Rana
7thBeing a parent, I saw my daughter improvement in her studies by seeing a good result in all day to day compititive exam TMO, NSO, IEO etc and as well as studies. I have got a fruitful result from my daughter.
Prisha Gupta
8thIt was good as the experience because as we had come here we had been improved in a such envirnment created here.Extra is taught which is beneficial for future.
Eshan Arora
8thIt was a good experience with Abhyas Academy. I even faced problems in starting but slowly and steadily overcomed. Especially reasoning classes helped me a lot.
Cheshta
10thAbhyas Methodology is very good. It is based on according to student and each child manages accordingly to its properly. Methodology has improved the abilities of students to shine them in future.
Manish Kumar
10thA marvelous experience with Abhyas. I am glad to share that my ward has achieved more than enough at the Ambala ABHYAS centre. Years have passed on and more and more he has gained. May the centre flourish and develop day by day by the grace of God.
Archit Segal
7thI have spent a wonderful time in Abhyas academy. It has made my reasoning more apt, English more stronger and Maths an interesting subject for me. It has given me a habbit of self studying
Yatharthi Sharma
10thAbhyas is a complete education Institute. Here extreme care is taken by teacher with the help of regular exam. Extra classes also conducted by the institute, if the student is weak.
Om Umang
10thAbout Abhyas metholodology the teachers are very nice and hardworking toward students.The Centre Head Mrs Anu Sethi is also a brilliant teacher.Abhyas has taught me how to overcome problems and has always taken my doubts and suppoeted me.
